三角比\(\sinθ,\cosθ,\tanθ\)にはどのような相互関係があるでしょうか。
・三角比の相互関係
半径1の半円の周上に点\(P(x,y)\)があるとき、\(\angle AOP=θ\) (\(0≦θ≦180°\)) とすると
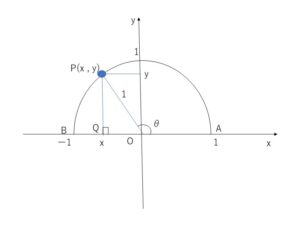
\(\sinθ=y\)・・・①
\(\cosθ=x\)・・・②
\(\tanθ=\displaystyle\frac{y}{x}\)・・・③
が成り立ちます。また三平方の定理より
\(x^2+y^2=1\)・・・④
が成り立ちます。(④は直角三角形\(OPQ\)ができない \((x,y)=(±1,0),(0,1)\) でも成り立つ。)
\(\cosθ≠0\) つまり \(θ≠90°\)のとき ①②を③に代入して
\(\tanθ=\displaystyle\frac{\sinθ}{\cosθ}\)・・・⑤
①②を④に代入すると
\(\sin^2θ+\cos^2θ=1\)・・・⑥
\(θ≠90°\)のとき ⑥の両辺を\(\cos^2θ\)で割ると
\(1+\tan^2θ=\displaystyle\frac{1}{\cos^2θ}\)・・・⑦
⑤~⑦は超重要公式です。必ず覚えましょう。
(問題1)
\(0°≦θ≦180°\) とする。
\(\sinθ=\displaystyle\frac{2}{5}\) のとき、\(\cosθ,\tanθ\) の値を求めよ。
(解答)
\(\sin^2θ+\cos^2θ=1\) より \(\cos^2θ=1-\sin^2θ\)
\(\cosθ=±\sqrt{1-\sin^2θ}=±\sqrt{1-\displaystyle\frac{4}{25}}=\)\(±\displaystyle\frac{\sqrt{21}}{5}\)
\(\tanθ=\displaystyle\frac{\sinθ}{\cosθ}=\displaystyle\frac{2}{5}÷(±\displaystyle\frac{\sqrt{21}}{5})=\)\(±\displaystyle\frac{2}{\sqrt{21}}\)
\(0°≦θ≦180°\) とする。
\(\tanθ=-2\) のとき、\(\sinθ,\cosθ\) の値を求めよ。
\(\tanθ=-2\) から、\(90°<θ<180°\) よって、\(\cosθ<0\)
\(\cos^2θ=\displaystyle\frac{1}{1+\tan^2θ}=\displaystyle\frac{1}{5}\)
\(\cosθ<0\)だから \(\cosθ=-\displaystyle\frac{1}{\sqrt{5}}\)
よって、\(\sinθ=-2・(-\displaystyle\frac{1}{\sqrt{5}})=\)\(\displaystyle\frac{2}{\sqrt{5}}\)
ここまで見て頂きありがとうございました。